The Phillips Curve & Aggregate Supply

Week 07

Vivaldo Mendes

vivaldo.mendes@iscte-iul.pt

Instituto Universitário de Lisboa (ISCTE-IUL)

October 27, 2025

Table of contents

• 1. The Phillips Curve
• 2. Shifts in the Phillips Curve
• 3. The Okun’s Law
• 4. The Aggregate Supply Curve (AS)
• 5. Readings

1. The Phillips Curve

The Pains of Fighting Inflation

“We have got to get inflation behind us. I wish there were a painless way to do that.” There isn’t .” Jerome Powel, Fed’s Chair, 21 Sept. 2022

The Phillips Curve (PC)

• Almarin Phillips discovered in the 1950s the kind of pain we have to suffer from reducing inflation.
• The PC shows how much more unemployment we will get \((\uparrow U)\), to reduce the inflation rate \((\downarrow \pi)\).
• The PC was easily confirmed in the 1960s.
• See next figure (click on any key) .

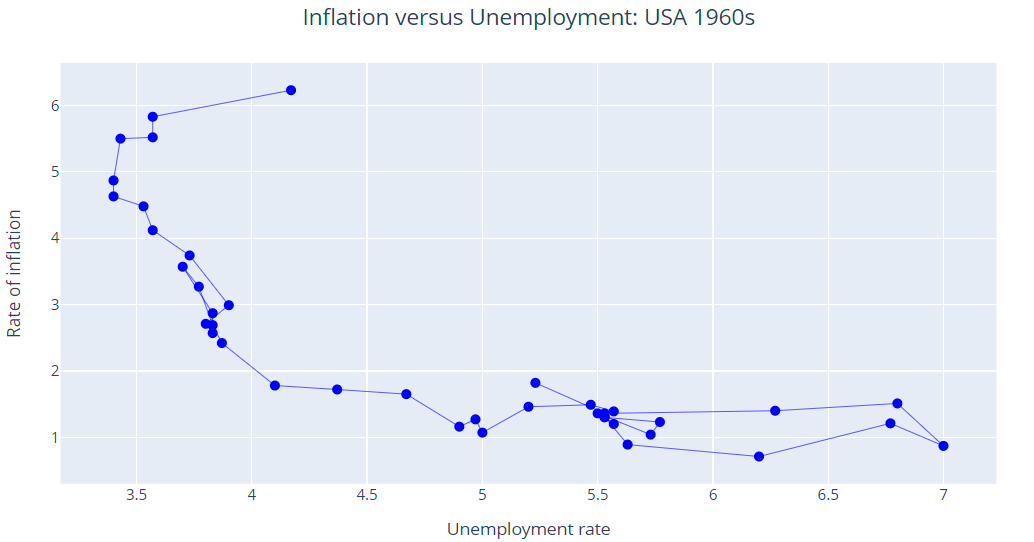
The Phillips Curve (PC)

In the 1960s, the PC was easy to spot and confirmed Phillips discovery.

The Phillips Curve in the 1970s

• In the 1970s, the PC begins to display a strange configuration: it seems to move constantly, looping around.

\(~~~~~\)

The Phillips Curve in the 1980s

• In the 1980s, the PC seems to have different slopes.

\(~~~~~\)

The Friedman-Phelps PC Curve

• Two Nobel prize winners – Milton Friedman and Edmund Phelps – showed how to write a PC that was able to explain those strange configurations.

• Following them, the expectations-augmented Phillips Curve should be written as:

\[ \pi=\pi^e-\omega\left(U-U^n\right) \tag{7.1} \]

• \(\pi\) inflation rate
• \(U\) unemployment rate
• \(\omega\) is a parameter

• \(\pi^e\) expected inflation rate
• \(\left(U-U^n\right)\) cyclical unemployment rate
• \(U^n\) natural unemployment rate

Oil Price Shocks

Large shocks in oil prices have been a recurrent major characteristic of the world economy since the early 1970s. They are temporary shocks.

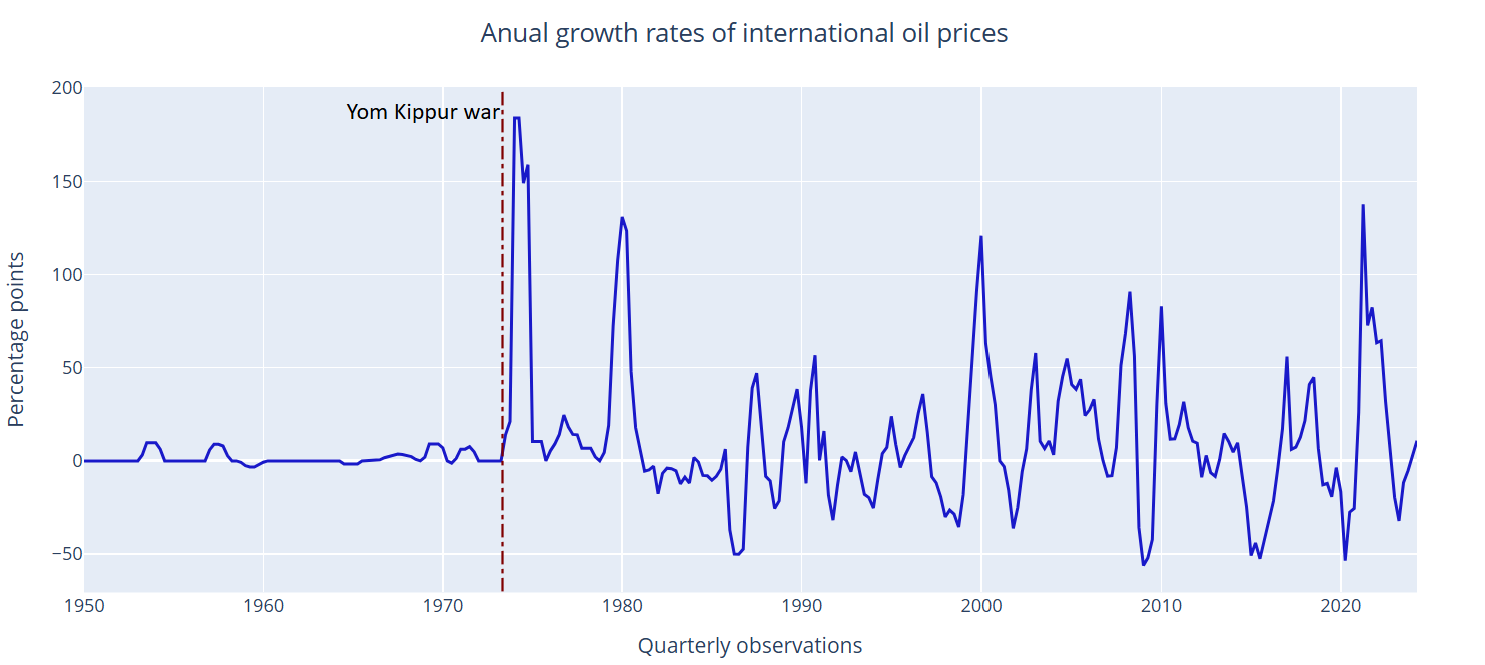
The PC with Supply Shocks

• Shocks in oil prices affect production costs and as such they interfere in the relationship between inflation and unemployment.
  • If oil prices increase a lot, production costs will also rise substantially, and inflation goes up, for every level of unemployment.
• The Covid19 pandemic has been a colossal shock on the supply side.
• The textbook calls these kind of shocks as temporary shocks: \[\color{red}{\rho}\]
• The PC can easily accommodate these temporary shocks:

\[ \pi=\pi^e-\omega\left(U-U^n\right)\class{fragment}{+ \color{red}{\rho}} \tag{7.2} \]

Inflation Expectations in the PC

• If inflation has been increasing recently, private agents expect that such trend will continue in the near future. A mathematical way of describing such a process is: \[\pi^e_t= \pi_{t-1} + \sigma(\pi_{t-1} - \pi_{t-2}) \tag{7.3}\]
• \(\pi^e_{t}\) is the inflation expectation for period \(t\).
• \(\pi_t\) and \(\pi_{t-1}\) are the inflation levels at periods \(t\) and \(t-1\).
• \(\sigma\) is a parameter of the degree of persistence of inflation expectations.
• To simplify the exposition, the textbook assumes that \(\sigma=0\), and we get: \[\pi^e_t= \pi_{t-1} \tag{7.4}\]

The PC with all Ingredients

The PC we will work with in this course includes three major ingredients:

1. Trending (or adaptive) expectations: \(\quad \color{red}{\pi^e=\pi_{-1}}\)
2. Cyclical unemployment: \(\quad \color{red}{\omega(U-U^n)}\)
3. Temporary supply shocks: \(\quad \color{red}{\rho}\)

Therefore, the final version of the PC is given by:

\[ \pi=\pi_{-1}-\omega\left(U-U^n\right)+\rho \]

The PC: Graphical Representation

\[\pi=\pi^e-\omega\left(U-U^n\right)+\rho \quad , \quad \color{black}{\pi^e=\pi_{-1}}\]

• If we want a lower unemployment rate \((\downarrow U)\)
• We have to accept a higher inflation rate \((\uparrow \pi)\)
• Assuming everything else constant \(\left(U^n, \pi^e, \rho\right)\).
• Graphically, this can be represented as in the next figure (click on any key)

The PC: Graphical Representation

\[\pi=\pi^e-\omega\left(U-U^n\right)+\rho \quad , \quad \color{black}{\pi^e=\pi_{-1}}\]

Example:

• \(\pi_{-1} = 2\%\),
• \(\omega=1.5\)
• \(U^n=5\%\)
• If \(U=4\%\)
• \(U<U^n \Rightarrow \uparrow \pi\)
• \(\pi=3.5\%\)

2. Shifts in the Phillips Curve

Shifts in the Phillips Curve \((\pi^e , \ \rho)\)

\[\pi=\pi^e-\omega\left(U-U^n\right)+\rho \quad , \quad \color{black}{\pi^e=\pi_{-1}}\]

• The Phillips Curve shifts when the following forces change:
  • Expected inflation (\(\pi^e\))
  • Supply shocks (\(\rho\))
  • Natural unemployment rate (\(U^n\))
• Let us concentrate on the first two forces (click on any key).

Shifts in the Phillips Curve \((\pi^e , \ \rho)\)

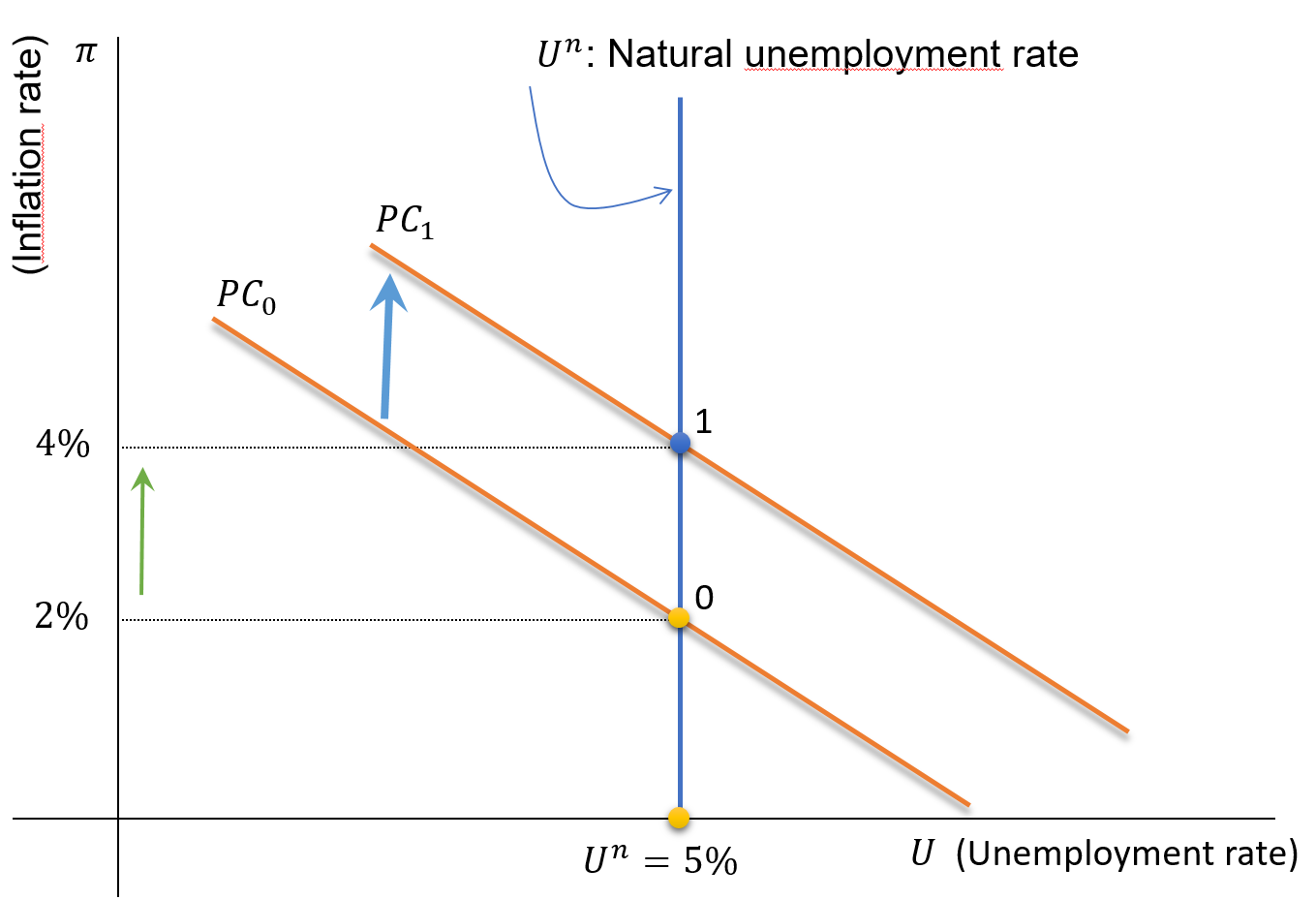
The PC shifts to the right if:

• \(\uparrow \pi^e\), \(~~\) or
• \(\uparrow \rho\)

Shifts in the Phillips Curve (\(U^n\))

\[\pi=\pi^e-\omega\left(U-U^n\right)+\rho \quad , \quad \color{black}{\pi^e=\pi_{-1}}\]

• The Phillips Curve shifts when the following forces change:
  • Expected inflation (\(\pi^e\))
  • Supply shocks (\(\rho\))
  • Natural unemployment rate (\(U^n\))
    
• Let us concentrate on the last force (click on any key).

Shifts in the Phillips Curve (\(U^n\))

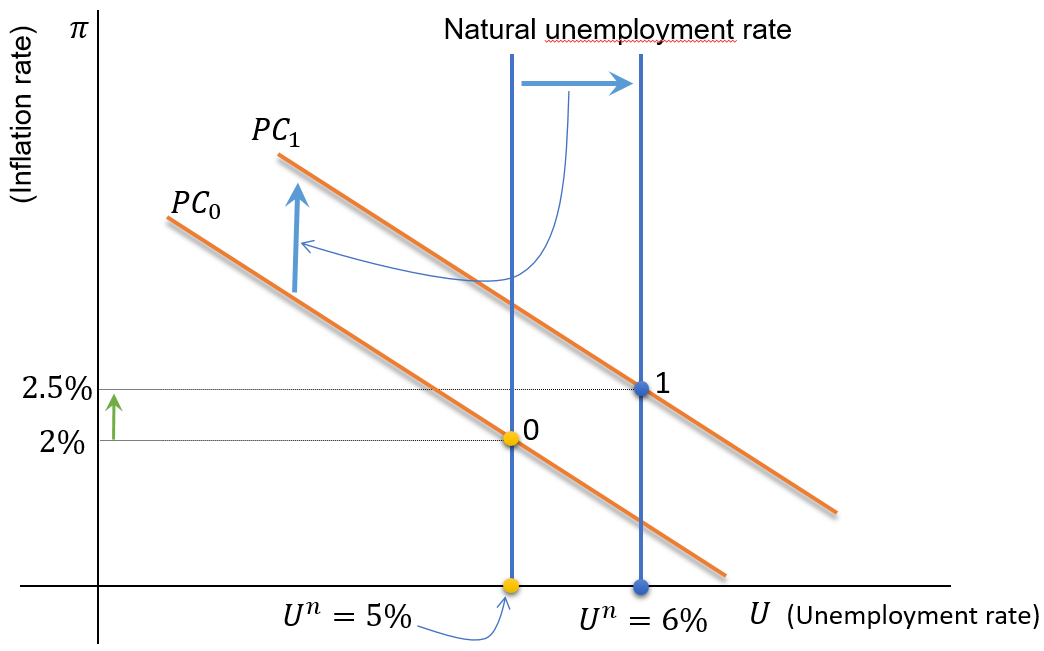
The PC will shift to the right if:

• \(\uparrow U^n\)
• Stable inflation will now be at 2.5%

Shifts in the PC: Inflationary Spiral Numerically

\[\pi=\pi^e-\omega\left(U-U^n\right)+\rho \quad , \quad \color{black}{\pi^e=\pi_{-1}}\]

• What happens if the government or the central bank try to keep the unemployment rate below the natural rate ?
  • \(U<U^n\)
    
  • The Phillips Curve will shift to the right
• Inflationary expectations : inflation will increase systematically over time

Shifts in the PC: Inflationary Spiral Numerically

\[\pi=\pi^e-\omega\left(U-U^n\right), \quad \omega=1.5, \quad \pi_t^e=\pi_{t-1}\]

• Suppose \(\pi _0 =2\%\)
• If, \(U_1<U^n_1\) Inflation will only stop if \(U\) returns to the \(U^n\) level
• But the result will be a higher \(\pi\) and the same initial \(U\)

Shifts in the PC: Inflationary Spiral Graphically

\[\pi=\pi^e-\omega\left(U-U^n\right), \quad \omega=1.5, \quad \pi_t^e=\pi_{t-1}\]

• The previous numerical example can be represented graphically
• Click on any key to continue

Shifts in the PC: Inflationary Spiral Graphically

\[\pi=\pi^e-\omega\left(U-U^n\right), \quad \omega=1.5, \quad \pi_t^e=\pi_{t-1}\]

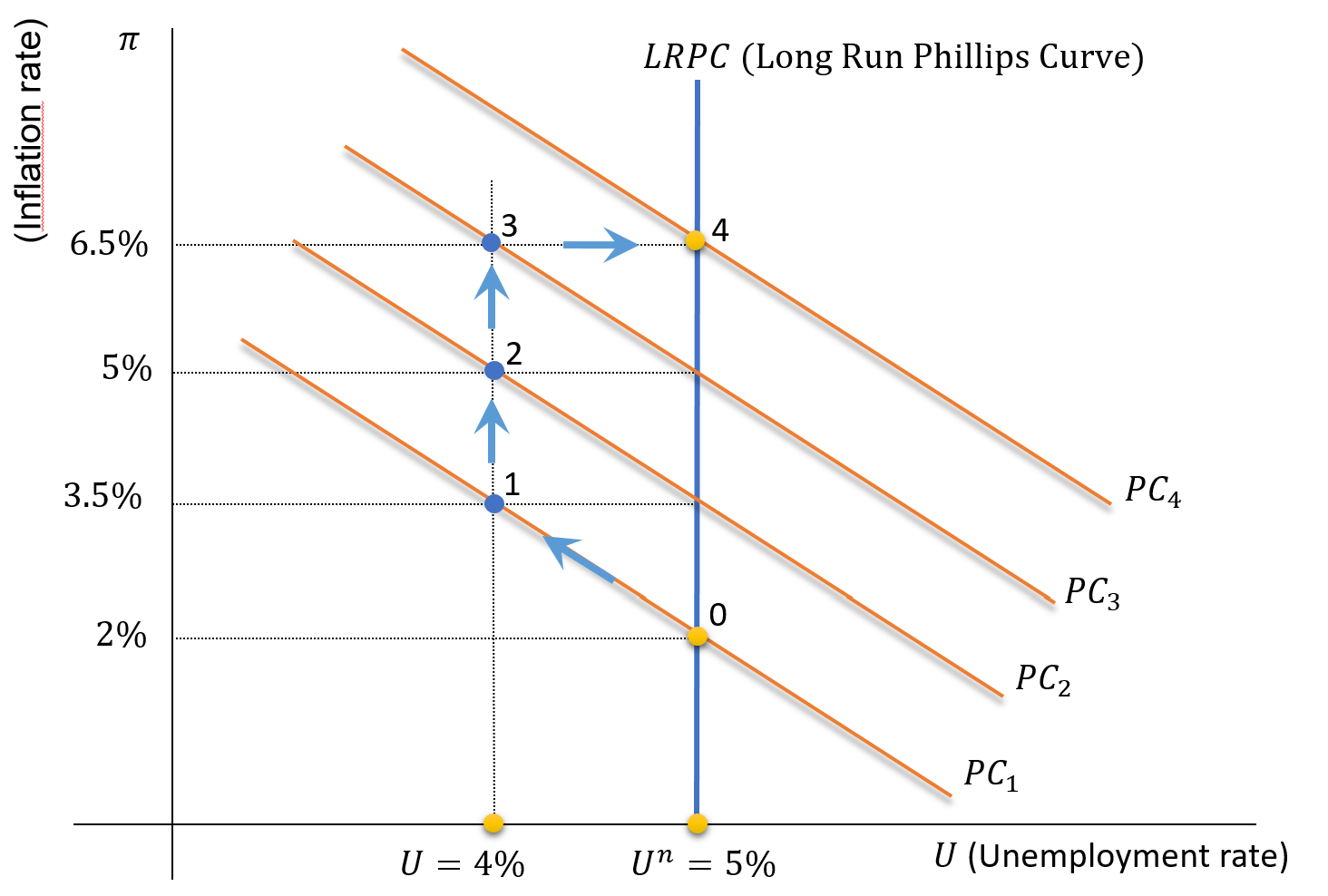
• Suppose \(\pi _0 =2\%\)
• Inflation will only stop if the \(U\) returns to the \(U^n\) level
• But the result will be a higher \(\pi\) and the same initial \(U\)

3. The Okun’s Law

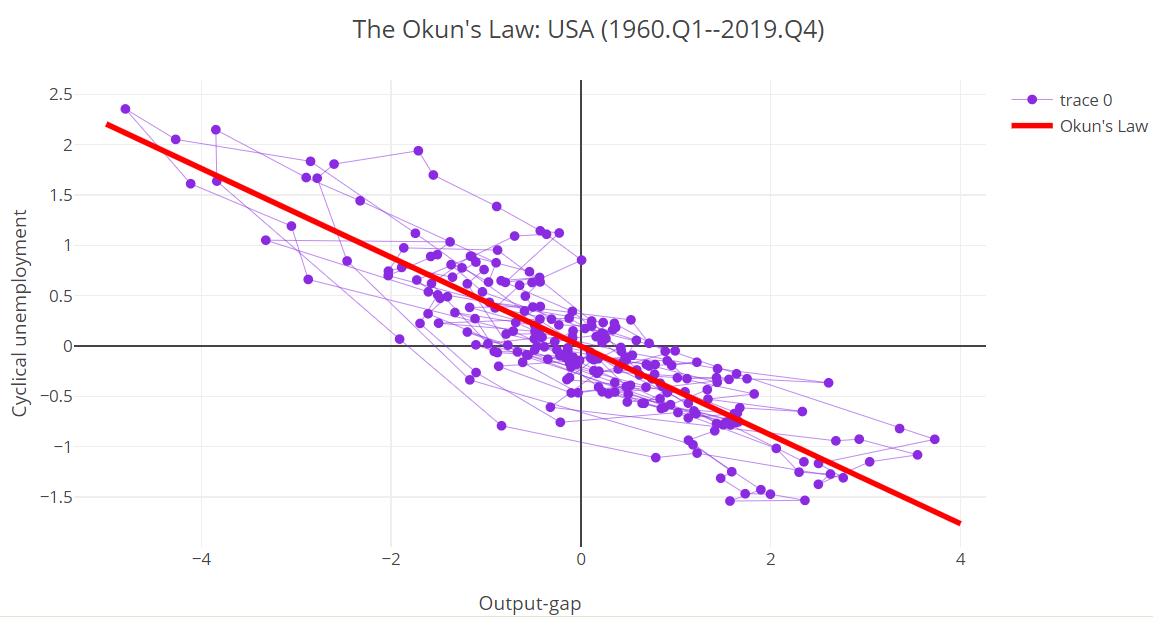
The Okun’s Law

• Arthur Okun showed in the early 1960s that there is a negative relationship between cyclical unemployment and the output-gap: \[ \underbrace{U-U^n}_{\text {Cyclical unemployment }}=-\theta \times \underbrace{\left(Y-Y^P\right)}_{\text {Output-gap }} \]

• where \(\theta\) is a parameter, for the USA economy usually close to: \[ \theta \simeq 0.5 \]

Arthur M. Okun (1962). “Potential GNP: Its Measurement and Significance”. Reprinted as Cowles Foundation Paper 190.

The Okun’s Law for the USA

The slope of the curve was \(−0.441\) for the period 1960-2019.

4. The Aggregate Supply Curve (AS)

The Short-Run AS Curve: Derivation

The Phillips Curve (PC): \[ \pi=\pi^e-\omega\left(U-U^n\right)+\rho \]

The Okun’s law: \[ U-U^n=-\theta \times\left(Y-Y^P\right) \]

• The short-run Aggregate Supply curve (AS) is obtained by inserting the Okun’s law into the Phillips Curve (PC). Therefore: \[ \pi=\pi^e-\omega \times \underbrace{\left[-\theta\left(Y-Y^P\right)\right]}_{=U-U^n}+\rho \tag{7.5} \]

• To simplify notation we will use: \(\gamma=\omega \theta\).

• So, the short-run AS curve is given by: \[ \pi=\pi^e+\gamma\left(Y-Y^P\right)+\rho \tag{7.6} \]

The AS Curve: Graphical Representation

\[ \pi=\pi^e+\gamma\left(Y-Y^P\right)+\rho \quad , \quad \pi^e = \pi_{-1} \]

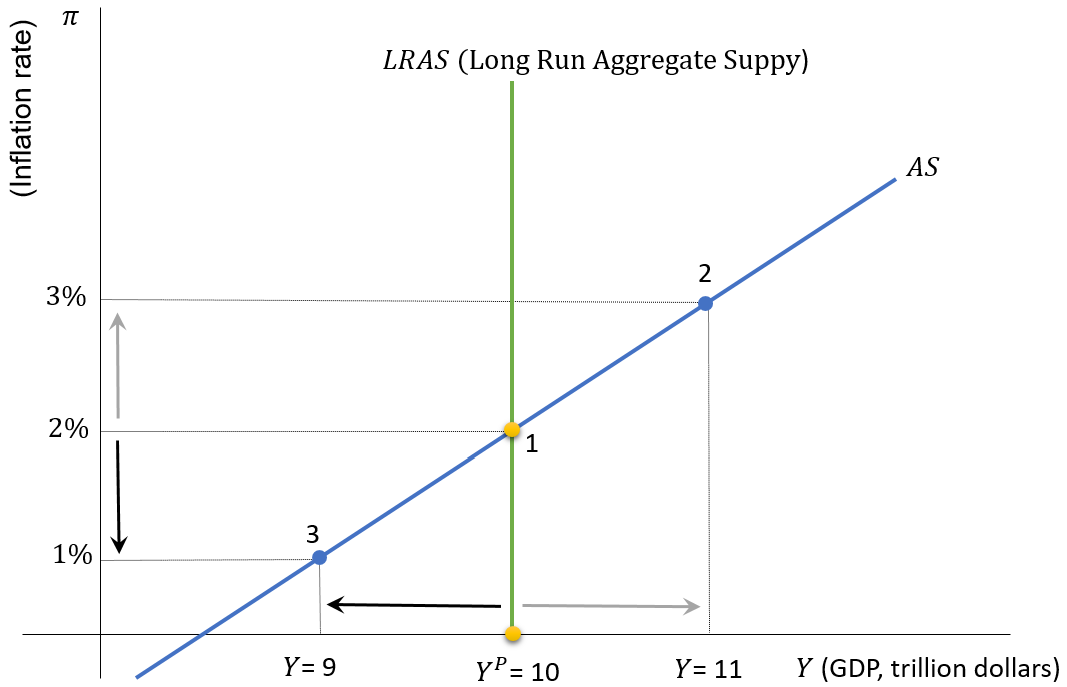
• In 1, \(Y=Y^P\)
• In 2, \(Y>Y^P\), economic boom, \(\pi\) increases to \(3\%\)
• In 3, \(Y<Y^P\), economic recession, \(\pi\) decreases to \(1\%\)

Shifts in the AS Curve (\(\pi^e, \rho\))

\[ \pi=\pi^e+\gamma\left(Y-Y^P\right)+\rho \quad , \quad \pi^e = \pi_{-1} \]

• The AS Curve shifts when the following forces change:
  • Expected inflation (\(\pi^e\))
  • Supply shocks (\(\rho\))
  • Potential GDP (\(Y^P\))
    
• Let us concentrate on the two first forces (click on any key).

Shifts in the AS Curve (\(\pi^e, \rho\))

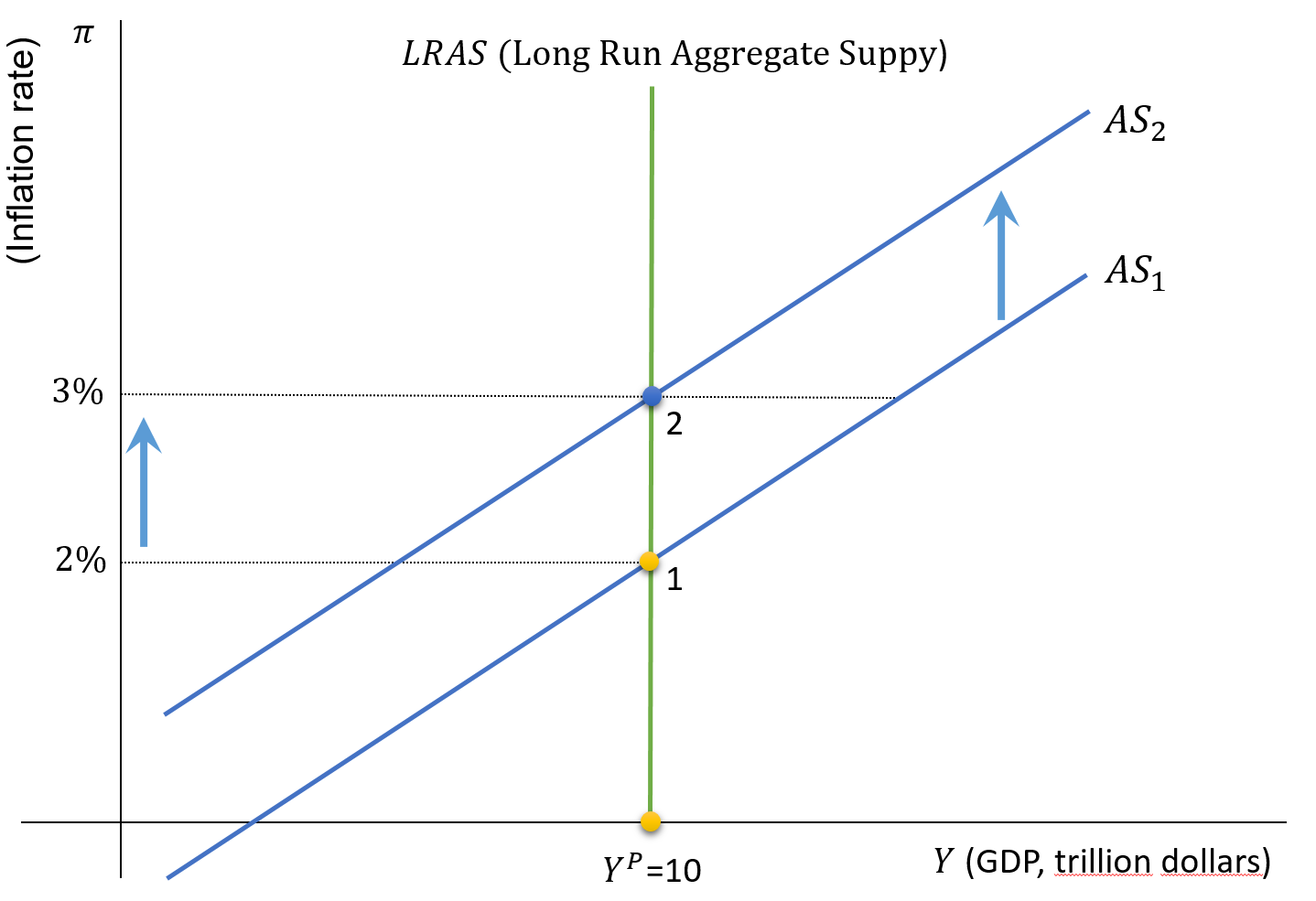
The AS shifts to the left if:

• \(\uparrow \pi^e\), or
• \(\uparrow \rho\)

Shifts in the AS Curve (\(Y^P\))

\[ \pi=\pi^e+\gamma\left(Y-Y^P\right)+\rho \quad , \quad \pi^e = \pi_{-1} \]

• The AS Curve shifts when the following forces change:
  • Expected inflation (\(\pi^e\))
  • Supply shocks (\(\rho\))]
  • Potential GDP (\(Y^P\))
• Let us concentrate on the last force (click on any key).

Shifts in the AS Curve (\(Y^P\))

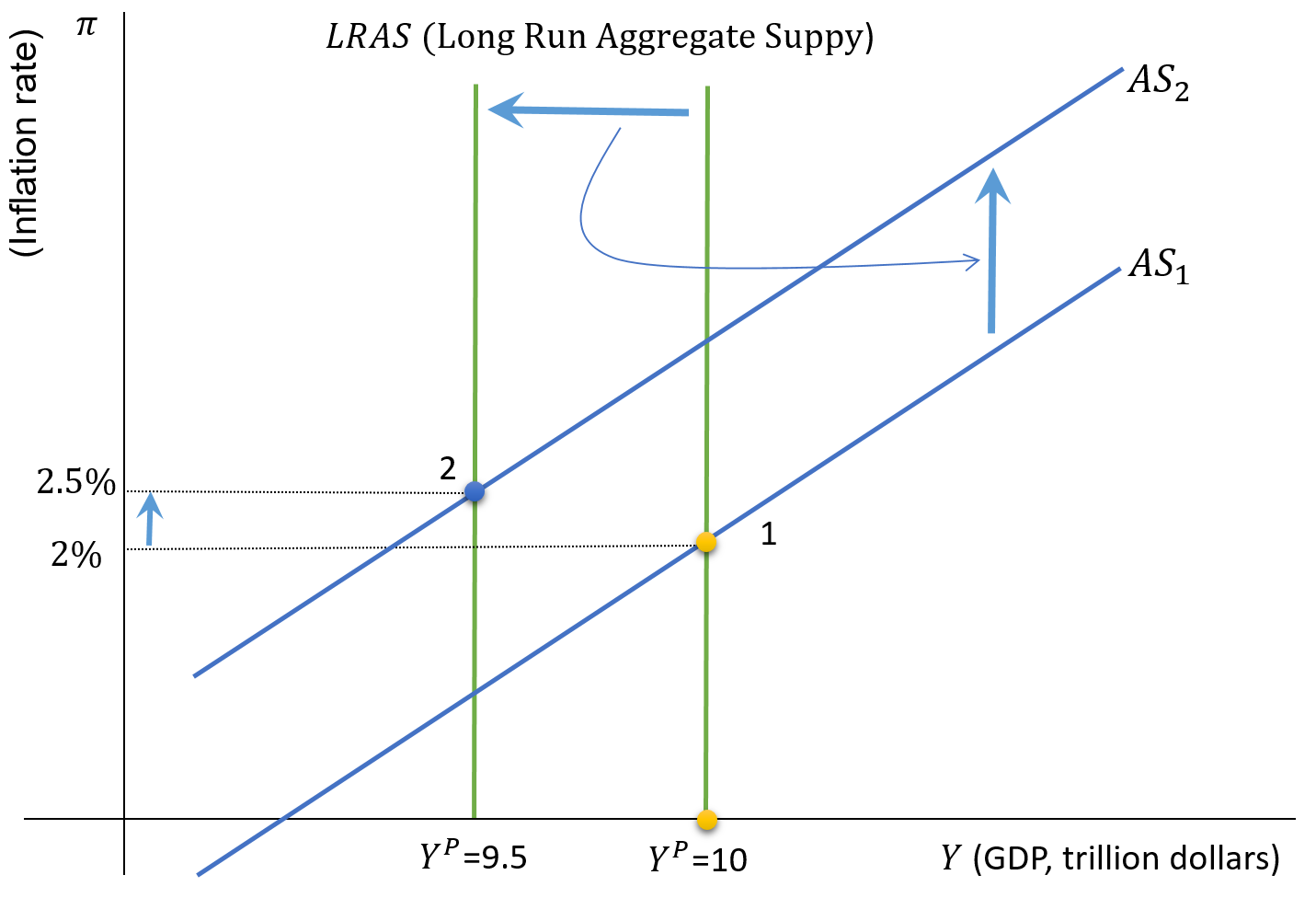
AS shifts to the left if:

• \(\downarrow Y^P\)
• The economy will have stable \(\pi\) only at point 2.
• At 2: \(\ \downarrow Y \ \) , \(\ \uparrow \pi\)

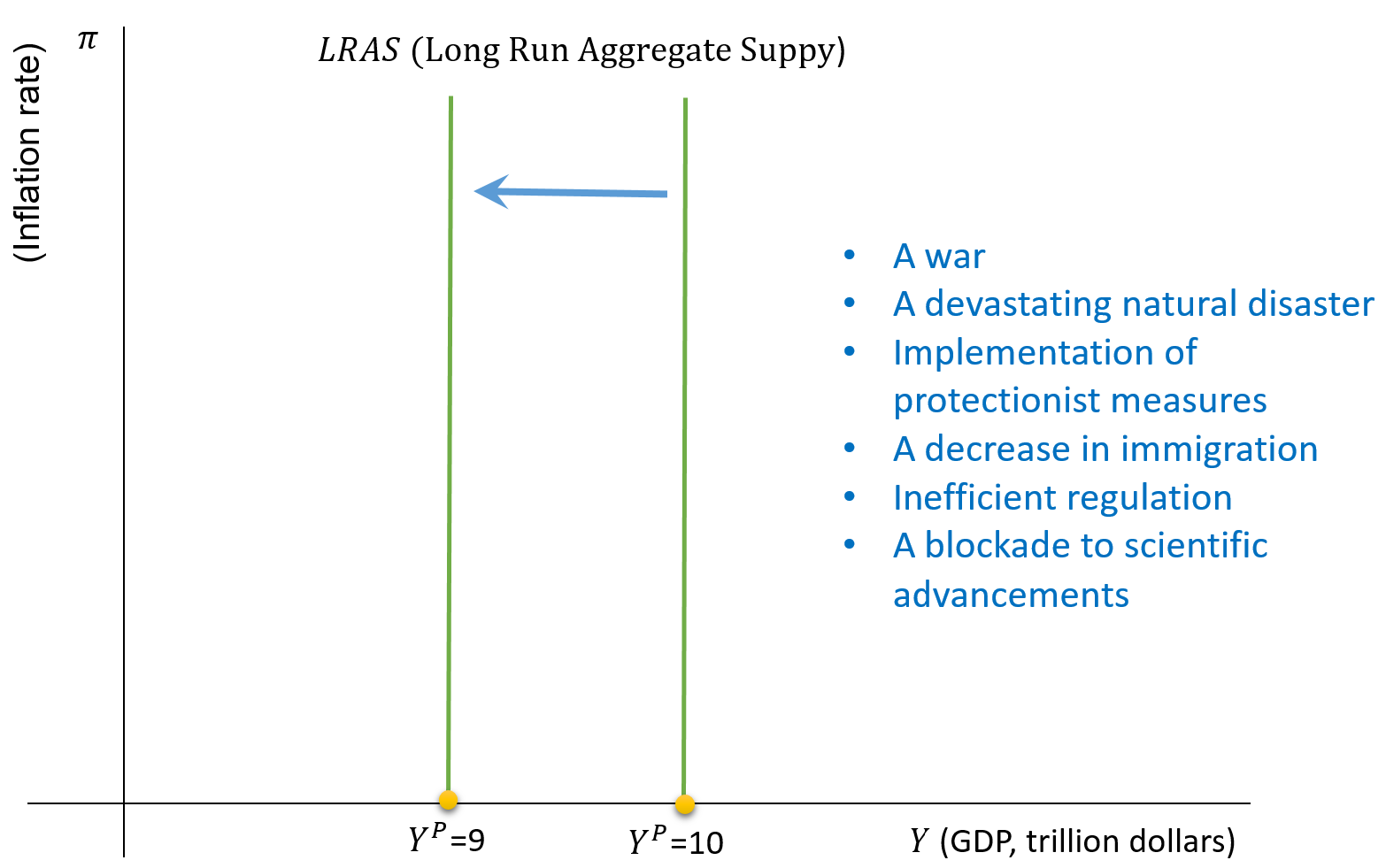
What Factors Shift the LRAS Curve?

• The factors that shift the LRAS curve are those that shift the production function studied in your Microeconomics course.
• The production function is given by: \[ Y=F(\cal{T}, K, L) \]
• The factors that shift the production function are:
  • \(\cal{T}\): Technology
  • \(\cal{ K}\): Capital
  • \(\cal{L}\): Labor

What Factors Shift the LRAS Curve?

Forces reducing any of the factors \(\{\cal{T}, K, L\}\) shift the LRAS to the left, and vice-versa.

5. Readings

Readings

Read Chapter 11 of the adopted textbook:

Frederic S. Mishkin (2015). Macroeconomics: Policy & Practice, Second Edition, Pearson Editors.